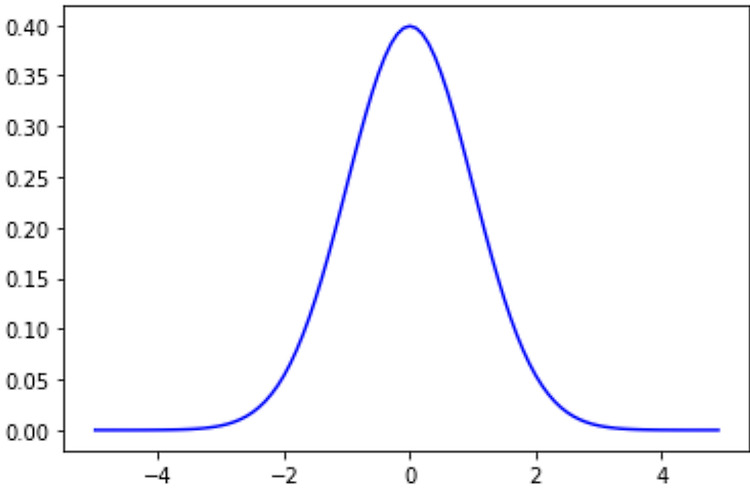
Normal DistirbutionGaussian Distribution, Laplace-Gaussian Distribution 라고도 불림.정의PDF는 다음과 같음. $$f(X)=\frac{1}{\sigma\sqrt{2\pi}}(e)^{-\frac{(X-\mu)^2}{2\sigma^2}}$$$\mu$ : mean$\sigma$ : standard deviation PDF 이므로 probability를 다 더한 적분값은 1이 됨.$\mu$와 $\sigma$가 parameter인데, 이들이 0과 1인 경우 standard normal distribution (표준정규분포)라고 불림.일반 normal distribution에 z-Transform을 수행하면 standard normal distribution..

가장 유명한 irrational number(무리수)로 대략 2.718281... 정도의 크기를 가짐.가장 유명한 극한값이기도 함: $0,1,\pi,e$ 는 가장 중요한 4가지 수라고도 불릴 정도이며 공학자에게 $e$는 정말 정말 중요함.Definition$$ \begin{aligned}e&=\lim_{n \to \infty}\left( 1+\frac{1}{n}\right)^n \\ &= \lim_{t \to 0} (1+t)^{\frac{1}{t}},\text{ where }t=\frac{1}{n} \end{aligned} $$주의할 것은$\frac{1}{n}$ (무한소에 해당)이 더해지고 이에 대한 reciprocal인 $n$ (무한대에 해당)이 exponent로 주어진다는 점임.더해지는 수와 expo..

Poisson Distribution이란? 아주 가끔 일어나는 사건(trial)에 대한 확률 분포 : 방사선 검출에 주로 사용되는 확률분포라 의료영상에서는 매우 많이 사용됨. 전체 인구수에서 연간 백혈병으로 사망 건수 특정 지역에서 발생하는 살인사건 건수나 군대의 연간 사망건수 Poisson distribution에서 평균 발생 건수(mean)가 $\lambda$인 경우에 $x$번 발생할 확률은 다음과 같음. (Poisson distribution의 PMF) $$ \text{Poisson}(x;\lambda)=\frac{\lambda^x e^{-\lambda}}{x!} $$ 이는 binomial distribution에서 $N$을 무한대로 보내고, mean이었던 $\mu=Np=\lambda$로 치환하여 ..

0. shifted impulse와 convolution은 결국 shifting 연산임$t_0$로 shifting을 시킨 impulse function $\delta(t-t_0)$과의 convolution은결국 같은 $t_0$만큼 signal을 shifting하는 것으로 볼 수 있음.$$f(t)*\delta(t-t_0) = f(t-t_0)$$$*$ : convolution1. 증명$$ \begin{aligned} f(t)* g(t) &= \int^\infty_{-\infty} f(t-\color{red}{\tau}) g(\tau) d \tau \\ f(t)* \delta(t-t_0) &= \int^\infty_{-\infty} f(t-\tau) \delta(\tau - t_0) d \tau \\ &= \..

CTFT 에서 Modulation Property란 $x(t)$의 CTFT가 $X(\Omega)$인 경우, $x(t) \cos (\Omega_0 t)$의 CTFT가 다음과 같음을 의미함. $$\mathcal{F}\left[ x(t) \cos (\Omega_0 t)\right] = \frac{1}{2} \left[ X(\Omega-\Omega_0) + X(\Omega+\Omega_0)\right]$$ 추가적으로, $x(t) \sin (\Omega_0 t)$의 CTFT도 다음과 같이 구해짐. $$\mathcal{F}\left[ x(t) \sin (\Omega_0 t) \right] = \frac{1}{2j} \left[ X(\Omega - \Omega_0) - X(\Omega + \Omega_0)\right..

Continuous Time Signal에서의 Impulse Function은 Dirac Delta Function $\delta(t)$임. 이는 다음을 만족함. $$\delta(t)=\left\{ \begin{matrix} \infty &,t=0 \\ 0 &,t \ne 0 \end{matrix}\right. \\ \int^\infty_{-\infty} \delta(t)dt=1$$ 2022.08.29 - [.../Signals and Systems] - [SS] Impulse Function (Dirac Delta Function) [SS] Impulse Function (Dirac Delta Function) 다음과 같이 정의 되는 함수를 $\delta_\epsilon(t)$라고 하자. $$\delta..