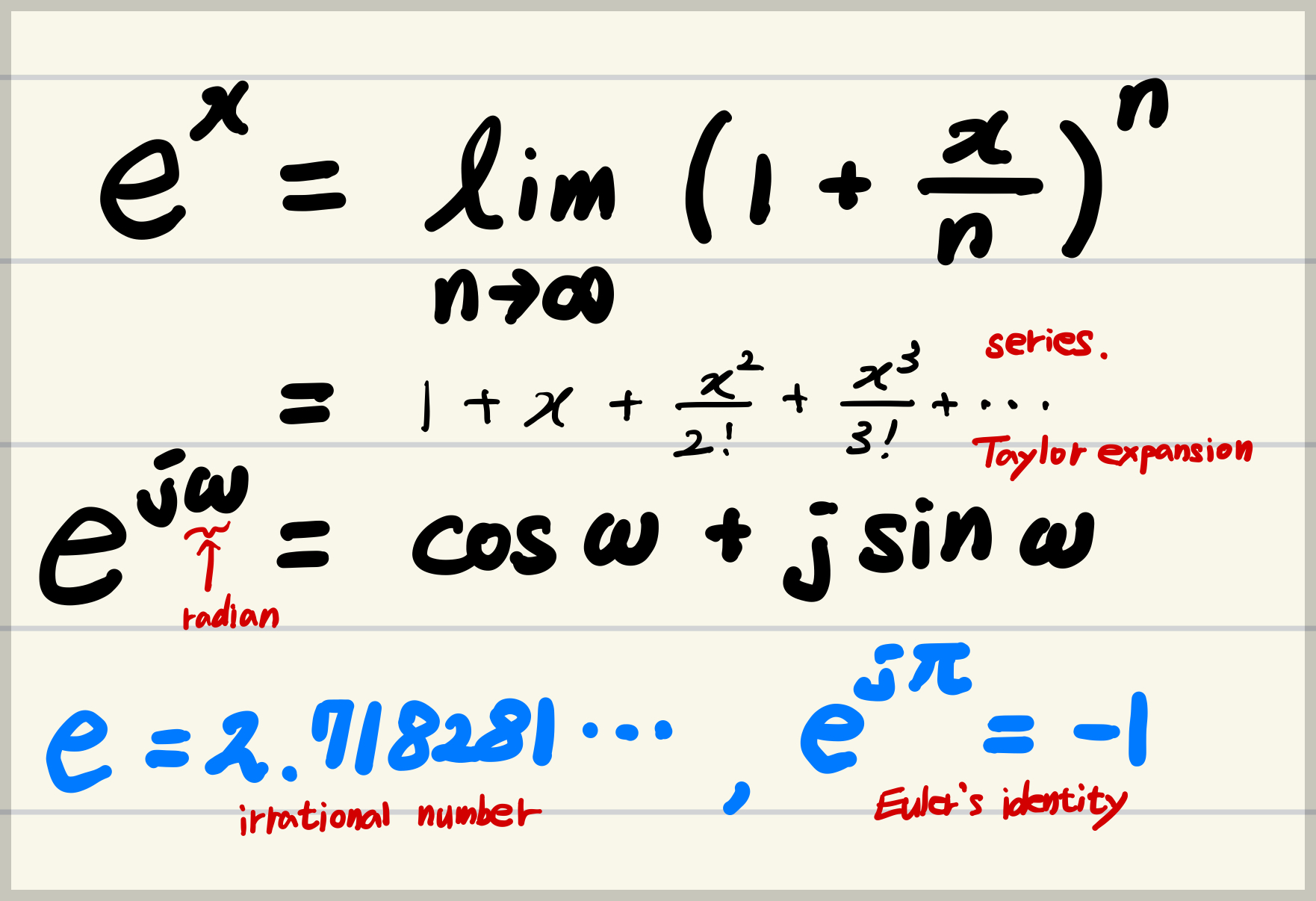
가장 유명한 irrational number(무리수)로 대략 2.718281... 정도의 크기를 가짐.
가장 유명한 극한값이기도 함: $0,1,\pi,e$ 는 가장 중요한 4가지 수라고도 불릴 정도이며 공학자에게 $e$는 정말 정말 중요함.
Definition
$$ \begin{aligned}e&=\lim_{n \to \infty}\left( 1+\frac{1}{n}\right)^n \\ &= \lim_{t \to 0} (1+t)^{\frac{1}{t}},\text{ where }t=\frac{1}{n} \end{aligned} $$
주의할 것은
- $\frac{1}{n}$ (무한소에 해당)이 더해지고 이에 대한 reciprocal인 $n$ (무한대에 해당)이 exponent로 주어진다는 점임.
- 더해지는 수와 exponent가 recipocal 이 성립해야 $e$가 되는 점을 기억할 것.
사실, 전기, 전자, 신호처리 등에서 Euler's Number (or Euler’s formula) 없이는 주파수 관련된 수식 대부분을 사용할 수 없다.
1에 무한소를 더하고 나서
더한 수의 reciprocal(역수)인 무한대로 제곱을 하면
바로 자연상수 $e$가 된다:
1의 무한대는 1인 것과 다름.
Taylor Expansion
2023.02.27 - [.../Math] - [Math] Taylor Expansion and Taylor Theorem (테일러 전개)
[Math] Taylor Expansion and Taylor Theorem (테일러 전개)
Taylor Expansion어떤 function $f(x)$을 : 주로 Trascedent Function 임어떤 point $a$에서의 값과 derivative들을 이용하여polynomial(다항식) $p(x)$으로 approximation(근사)하는데 사용되는 것이바로 Taylor's Expansion이라고
dsaint31.tistory.com
암기(?)용 동영상
https://youtu.be/jcSi_uVrpSs?si=YiQRqVJpM1iVspgD
주요 성질
$$ \begin{aligned}e^x &= \underset{n \to \infty}{\lim} \left( 1+\frac{x}{n}\right)^n \\ &= 1+x + \frac{x^2}{2!}+\frac{x^3}{3!} +\dots \end{aligned} $$
- 위의 등식은 정의에 의해서,
- 아래의 등식은 Taylor's Expansion 에 의해
Euler's Identity
이 Euler Number를 이용한 Euler's identity : Trigonometric functions 와 complex exponential functions를 이어줌.
$$ e^{j\omega}=\cos \omega +j \sin\omega \\ e^{j\pi}+1=0 $$
- $x$ : real number.
- $j=\sqrt{-1}$ : imaginary number
- $w$ : radian 임.
Poisson Distribution에서의 사용.
binomial distribution에서 Poisson distribution을 유도할 때, 다음의 전개과정이 사용됨.
- $\lambda$ : Poisson distribution의 mean / binomial distribution의 $Np$
- $N$ : binomial distribution에서의 Bernoulli trial 시행횟수
$$\begin{aligned}\lim_{n \to \infty} \left( 1-\frac{\lambda}{n}\right)^n &=\lim_{n \to \infty} \left( 1 +\left(-\frac{\lambda}{n}\right)\right)^n\\&=\lim_{n \to \infty} \left( 1 +\left(-\frac{\lambda}{n}\right)\right)^{-n\frac{-\lambda}{\lambda}}\\&=\lim_{n \to \infty} \left( 1 +\left(-\frac{\lambda}{n}\right)\right)^{-\frac{n}{\lambda}(-\lambda)}\\ &= \lim_{t \to 0} \left(1+\frac{1}{t}\right)^{t(-\lambda)},\text{ where }t=-\frac{n}{\lambda}\\&=e^{-\lambda}\end{aligned}$$
2023.03.14 - [.../Math] - [Math] Binomial Distribution (이항분포)
[Math] Binomial Distribution (이항분포)
1. 정의 1이 나올 확률(or 성공확률)이 $p$이고, 0이 나올 확률(or 실패확률)이 $1-p$인 Bernoulli trial을 $N$번 반복하는 경우의 성공횟수를 Random Variable $X$라고 할 경우, $X$가 따르는 probability distribution(확
dsaint31.tistory.com
신호처리에서의 활용 : complex expontial signal
2023.09.19 - [.../Signals and Systems] - [SS] Complex Exponential Signals
[SS] Complex Exponential Signals
Continuous-time Sinusoidal Signal 주기신호 Continuous-time sinusoidal signal은 특정 angulary frequency $\omega$와 phase $\phi$, 그리고 amplitude $A$로 정의된다. $$ A\sin (\omega t+\phi) \\ A\cos(\omega t+ \phi)$$ 이를 exponent가 imaginar
dsaint31.tistory.com
'... > Math' 카테고리의 다른 글
| [Math] Cartesian Product (or Descartes Product, Product Set) (0) | 2024.02.04 |
|---|---|
| [Math] Normal Distribution (정규분포, Gaussian Distribution) (2) | 2023.10.25 |
| [Math] Poisson Distribution (포아송분포) (2) | 2023.10.25 |
| [Math] Derivative of Logistic Function (0) | 2023.09.25 |
| [Math] Error Function (0) | 2023.09.24 |