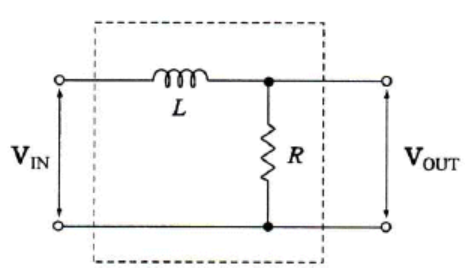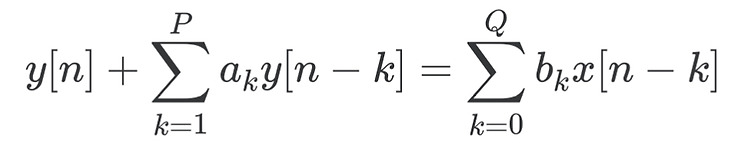
[SS] Discrete System Representation: DE, MA, AR, ARMA, ARIMA, SARIMA
·
.../Signals and Systems
신호처리 관점에서의 시스템 표현: 차분 방정식과 MA, AR, ARMA, ARIMA, SARIMA 모델신호처리에서 이산 신호 시스템은 FIR(Finite Impulse Response) 시스템과 IIR(Infinite Impulse Response) 시스템으로 분류할 수 있음.이들은 difference equation(차분 방정식)을 통해 수학적으로 표현가능함.그러나 차분 방정식만으로는 신호의 주파수 응답, 자기 상관성, 노이즈 제거 등의 다양한 특성을 효과적으로 반영한 모델링이 어려움. 이를 보완하기 위해 다음의 확장된 형태의 표현(representation, model)이 도입됨.MA(Moving Average),AR(Auto-Regressive),ARMA(Auto-Regressive Moving A..