[Math] Limit Laws of Multivariate Function

1. Limit Laws

Assume that $S\subseteq \mathbb{R}^n$ and that $\textbf{a}$ is a point in $\textbf{R}^n$ is a limit point of $S$
(for example, an interior point of $S$).
Further assume that $f,g:S\to \mathbb{R}$ are functions and
$L$,$M$ are numbers such that

$$\lim_{\mathbf x \to \mathbf a}f(\mathbf x) = L,
\qquad
\lim_{\mathbf x \to \mathbf a}g(\mathbf x) = M.$$

 

Then

$$\lim_{\textbf x \to \textbf a}[f(\textbf x)+ g(\textbf x)] = L+M, \\\\ \lim_{\textbf x \to \textbf a}[f(\textbf x) g(\textbf x)] = LM, \\\\ \displaystyle \frac{\lim_{\textbf x \to \textbf a}f(\textbf{x})}{\lim_{\textbf x \to \textbf a}g(\textbf{x})}=\frac{L}{M},\text{ where } \lim_{\textbf x \to \textbf a}g(\textbf{x}) \ne 0 \\\\ \lim_{\textbf x \to \textbf a}cf(\textbf{x}) = c\lim_{\textbf x \to \textbf a}f(\textbf{x}) \text{ and } \lim_{\textbf x \to \textbf a}cg(\textbf{x}) = c\lim_{\textbf x \to \textbf a}g(\textbf{x}) \text{ ,where } c \text{ is a constant scalar} $$

---

참고 : Limit Point

The point $\textbf{a} \in \mathbb{R}^n$ is a limit point of set $S$ if and only if

$$\begin{equation}\label{limitpoint} \forall \delta>0, \quad
\exists \mathbf x\in S \quad\mbox{ such that }\quad
0 < |\mathbf x - \mathbf a|<\delta.
\end{equation}$$

 

https://dsaint31.tistory.com/536#Definition%--of%--Limit%--point

[Math] Limit of a multi-variate function and Limit Point

---

2. Squeeze Theorem (샌드위치 정리)

Assume that $S\subseteq \mathbb{R}^n$ and
that $\textbf{a}$ is a point in $\textbf{R}^n$ is a limit point of $S$ (for example, an interior point of $S$).
Further assume that $f,g,h:S\to \mathbb{R}$ are functions and
there exists real numbers $\delta > 0$ and $L$ such that

$$f(\mathbf x)\le g(\mathbf x) \le h(\mathbf x)\mbox{ for all }\mathbf x\in S \mbox{ such that }0<|\mathbf x-\mathbf a|<\delta$$

and

$$\lim_{\mathbf x \to \mathbf a}f(\mathbf x) = \lim_{\mathbf x\to \mathbf a}h(\mathbf x) = L.$$

Then

$$\lim_{\mathbf x\to \mathbf a}g(\mathbf x) = L$$.

---

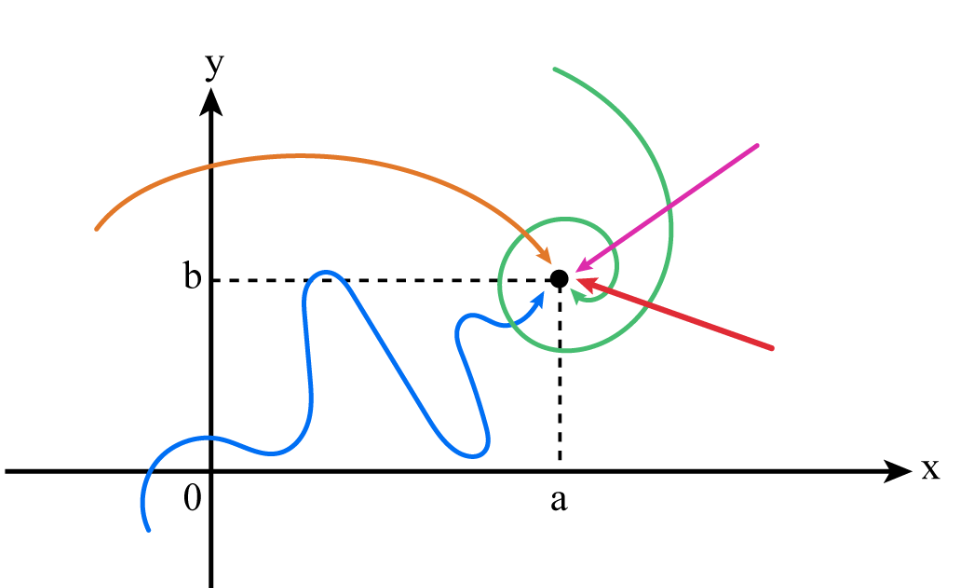
3. 극한의 존재 (converge)

Limit이 존재할 때 → 수렴(Converge)한다 고 말함.

Limit이 존재하지 않을 시 → 발산(Diverge)한다 고 말함.

 

limit이 존재할 경우 아래와 같이 서로 다른 경로를 취하더라도 동일한 limit을 가지게 됨.
(scalar function에서는 left side limit과 right side limit이 같음을 보이면 되었던 것이 확장되었음)

original :&nbsp;https://calcworkshop.com/partial-derivatives/multivariable-limits/

 

앞서 다룬 squeeze theorem은 주로 limit이 존재함을 증명하는데 사용된다.

반면, limit이 존재하지 않는 것을 증명하려면, 극한값이 다른 2개의 경로를 찾으면 됨.

 

scalar function에서의 극한의 존재.

2024.02.27 - [.../Math] - [Math] Limit of Scalar Function: Left-sided Limit and Right-sided Limit

[Math] Limit of Scalar Function: Left-sided Limit and Right-sided Limit