Geometric Camera Model (or Camera Model)은
- real world 와 camera의 pose에 따라,
- real world 와 camera의 image 간의 관계를
- approximation 함.
이 문서에서는 기본적인 Pinhole Camera Model에 기반하여 설명함.
- pinhole camera는 매우 기본적인 image acquisition의 수단임.
- 초점이 잘 맺어진 선명한 상을 얻기 위한 가장 쉬운 방법이지만,
- light이 작은 구멍(pinhole)을 통해 들어오기 때문에 매우 긴 촬영시간 (~노출시간, exposure time)이 필요함.
- 때문에 현재의 카메라는 모두 lens기반임.
2024.08.09 - [Programming/DIP] - [CV] Ideal Pinhole Size
[CV] Ideal Pinhole Size
Pinhole Camera는 가장 기본적인 Camera Model로 Perspective Projection을 통해 image가 얻어진다. 보통 pinhole은 아주 작은 diameter의 구멍이지만, 사용하는 빛의 파장과 effective focal length에 따라 최적의 image를
dsaint31.tistory.com
1. Pinhole Camera Model
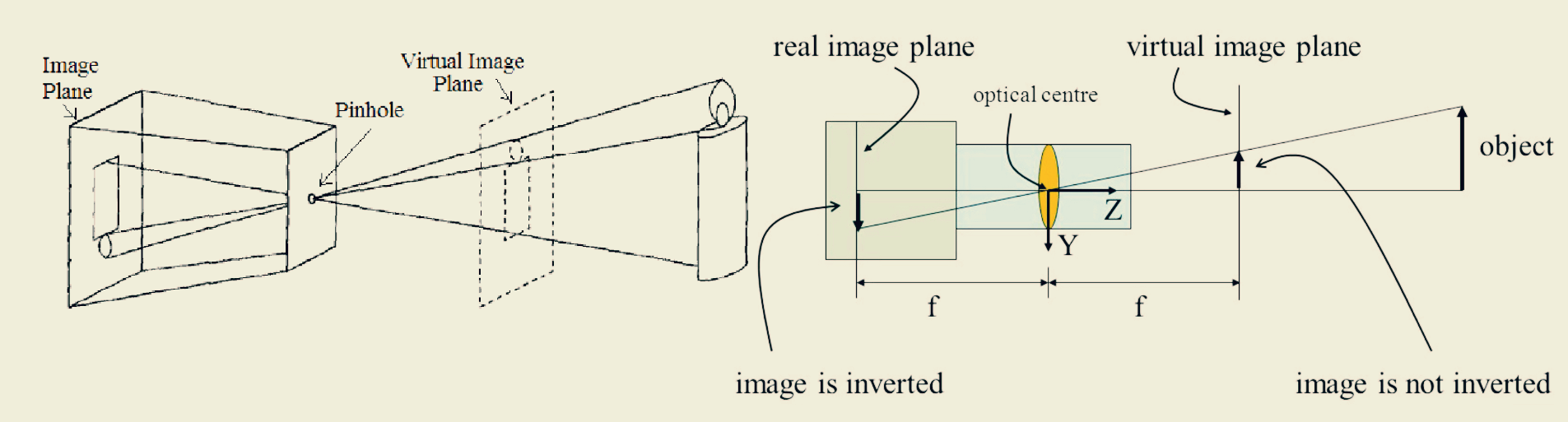
핀홀 카메라에서는 빛이 직선으로 이동하기 때문에, 외부의 물체는 이미지 평면에 거꾸로 된 상을 형성하게 됨.
- Pinhole (핀홀): 작은 구멍을 통해 외부의 빛이 들어와서 이미지 평면에 상을 맺음.
- Virtual Image Plane (가상 이미지 평면): 실제 이미지 평면의 반대편에 위치하며, 가상 이미지를 형성하는 평면.
- 뒤집혀 있는 (real) image plane 대신 사용되며 같은 좌표계들을 적용함.
- (Real) Image Plane (이미지 평면):
- 빛이 핀홀을 통해 들어와서 실제 이미지를 형성하는 평면
- x-y 좌표계: image coordinate
- 카메라의 image sensor 표면의 좌표계로 image coordinate라고도 불림.
- 센서의 셀 크기 등을 반영하여 $mm$ 또는 $\mu m$ 등의 물리적 단위로 표현됨.
- u-v 좌표계: pixel coordinate
- image의 pixel 단위를 사용하는 좌표계.
- sensor coordinate 가 적용되며, left top을 origin으로 삼음.
- 카메라의 intrinsic parameters로 구성된 intrinsic matrix $K$를 comera coordinate에 적용하여 얻어냄.
2024.07.06 - [Programming/DIP] - [CV] Image Plane to Image Sensor Mapping
[CV] Image Plane to Image Sensor Mapping
Image Plane에서의 coordinate $x_i, y_i$는mm단위를 가지며결과 image의 coordinate라고 생각할 수 있다.하지만 계산의 용이성 때문에 $x_i, y_i$ 보다는normalized image plane의 coordinate $x_n, y_n$ 이 많이 사용된다.현
dsaint31.tistory.com
2024.06.29 - [Programming/DIP] - [CV] Coordinate Systems
[CV] Coordinate Systems
이 글은 Computer Vision에서의 Coordinate Systems 을 다룬다.종류 및 정의World Coordinate (월드 좌표계):$\begin{bmatrix}x_w& y_w & z_w & 1\end{bmatrix}^T$카메라 외부에 존재하는 객체(object)의 위치를 전역적인 좌표계
dsaint31.tistory.com
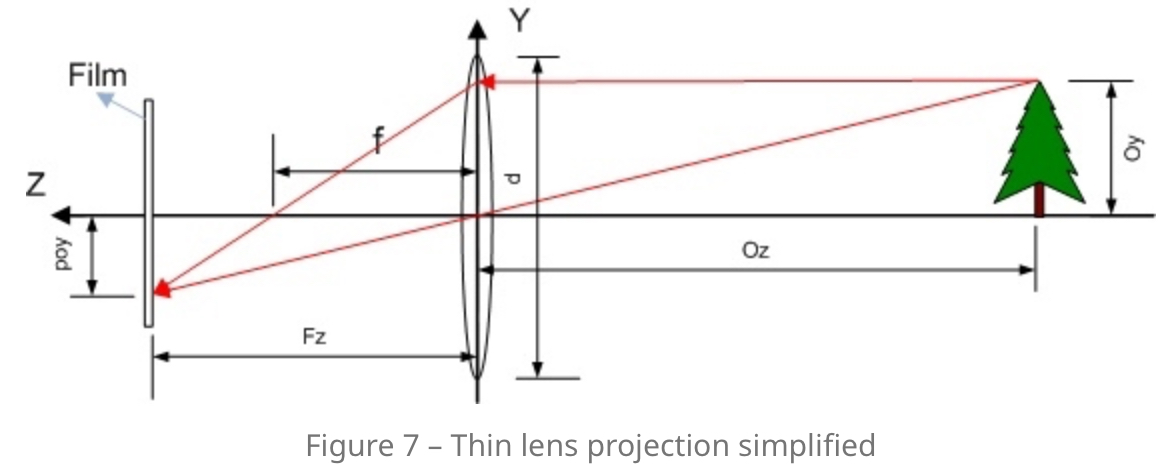
다음은 lens를 사용하는 경우에 대한 모식도임.
참고: Thin Lens Camera Model
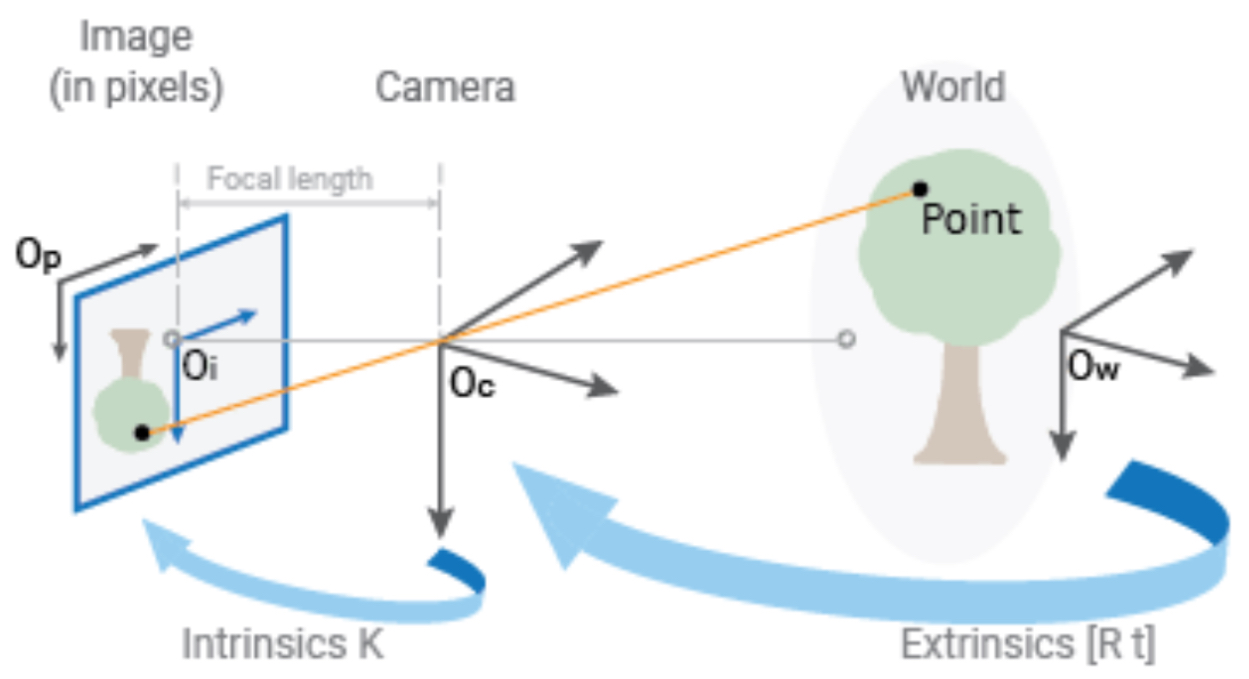
2. Parameters: Intrinsic and Extrinsic
Real world의 좌표계와 Camera의 좌표계, 그리고 이미지의 좌표계간의
관계를 Camera Model은 approximation 하며,
다음의 파라메터로 구성됨.
2-1. Intrinsic Parameters (Camera가 내재적으로 가지는 parameters)
- Principal Point, $\color{red}{\begin{bmatrix}u_o,v_o\end{bmatrix}^\top}$ : optical center
- Focal Length : $\color{red}{f_x,f_y}$ : unit aspect ratio $f_x=f_y$
- $f_x = m_x f, f_y = m_y f$ : $m_x, m_y$ are pixel densities (pixels/mm)
- Skew Coefficient (최근의 digital camera에서 0임), $\color{red}{s}$ : no skew $s=0$
- camera intrinsic matrix $K$로 표현된다.
Extrinsic Parameters는
- World coordinate 를 Camear Coordinate로 바꾸고
Intrinsic Parameters는
- depth $z_c$가 1이 되도록 normalization 시킨 normalized image plane의 좌표 $\begin{bmatrix}x_n & y_n & 1\end{bmatrix}^\top$를
- 실제 image 상의 좌표 $\begin{bmatrix}u & v & 1\end{bmatrix}^\top$로 바꾸어주는 역할을 한다.
$$\begin{bmatrix}u \\ v \\ 1\end{bmatrix} = K \begin{bmatrix} x_n \\y_n \\1 \end{bmatrix} = \begin{bmatrix} f_x & s & u_o \\ 0 & f_y & v_o \\ 0 & 0 & 1 \end{bmatrix}\begin{bmatrix} x_n \\y_n \\1 \end{bmatrix}$$
오늘날 카메라는 대부분 unit aspect ratio라 $f_x = f_y=f$이고, skew 가 없다($s=0$).
때문에 다음의 관계가 성립함
$$ x_n = \frac{u-u_o}{f} = \frac{x_c}{z_c} \\ y_n = \frac{v-v_o}{f} = \frac{y_c}{z_c} $$
Camera Calibration에서 $K$는 normalized image plane의 $\begin{bmatrix} x_n & y_n & 1 \end{bmatrix}^\top$를 구하기 위해 $K^{-1}$로 사용된다.
즉, normalized image plane의 좌표는 camera calibration이 적용된 결과임.
2-2. Extrinsic Parameters (Real world에서 Camera의 pose에 의해 결정되는 parameters)
- Camera Position, $\color{blue}{\textbf{t}}$
- Camera Orientation, $\mathbf{R}$ : camera rotation matrix (Orthogonal Matrix임)
2022.11.17 - [.../Math] - [LA] Orthogonal matrix (직교행렬)
[LA] Orthogonal matrix (직교행렬)
Orthogonal MatrixMatrix의 row vector (and column vector)들이자기자신을 제외한 나머지 row vector (and column vector)들과 orthonormal인 square matrix.$$A^{-1}=A^T \\A^TA = I \\\mathbf{a}_i^T \mathbf{a}_j = \mathbf{a}_i \cdot \mathbf{a}_j = 0
dsaint31.tistory.com
Projection from 3D world coordinates to 2D image coordinates
$$w\begin{bmatrix}u \\ v \\ 1 \end{bmatrix} = \color{red}{\mathbf{K}} \begin{bmatrix} \mathbf{R} & \color{blue}{\textbf{t}} \end{bmatrix} \begin{bmatrix} x \\ y \\ z \\ 1 \end{bmatrix} = \color{red}{\begin{bmatrix} f_x & s & u_o \\ 0 & f_y & v_o \\ 0 & 0 & 1 \end{bmatrix}} \begin{bmatrix} r_{11} & r_{12} & r_{13} & \color{blue}{t_x} \\ r_{21} & r_{22} & r_{23} & \color{blue}{t_y} \\ r_{31} & r_{32} & r_{33} & \color{blue}{t_z}\end{bmatrix} \begin{bmatrix} x \\ y \\ z\\ 1 \end{bmatrix} $$
World Coordinate를 Camera Coordinate로 변경해주는 것이 Extrinsic Parameters로 구성된 Extrinsic Matrix $\begin{bmatrix}R & t\end{bmatrix}$가 하는 역할.
3. DoF
위의 camera model의 parameter는 결국 11개임 (Degree of Freedom=11)
- $\color{red}{\mathbf{K}}$ : DoF=5
- $\mathbf{R}=\mathbf{R}_z\mathbf{R}_y\mathbf{R}_x=\begin{bmatrix} \cos \theta_z & - \sin\theta_z & 0 \\ \sin\theta_z & \cos \theta_z & 0 \\ 0 & 0 & 1 \end{bmatrix}\begin{bmatrix} \cos \theta_y & 0 & -\sin\theta_y \\ 0 & 1 & 0 \\ \sin\theta_y & 0 & \cos\theta_y \end{bmatrix}\begin{bmatrix} 1 & 0 & 0 \\ 0 & \cos \theta_x & -\sin \theta_x \\ 0 & \sin \theta_x & \cos \theta_x \end{bmatrix}=\begin{bmatrix} \mathbf{x-axis}_w^\top \\ \mathbf{y-axis}_w^\top \\ \mathbf{z-axis}_w^\top\end{bmatrix}$ : DoF=3
- $\mathbf{x-axis}_w, \mathbf{y-axis}_w, \mathbf{z-axis}_w$은
- camera coordinate system (=camera frame)의 각 축의 방향을 나타내는
- unit vector $\hat{\mathbf{x}}_c, \hat{\mathbf{y}}_c, \hat{\mathbf{z}}_c$를
- world coordinate system 으로 나타낸 것임.
- 즉, "world frame"에서 카메라 좌표계의 각 축의 방향을 나타내는 unit vector임.
- $\mathbf{x-axis}_w, \mathbf{y-axis}_w, \mathbf{z-axis}_w$은
- $\color{blue}{\textbf{t}}$ : DoF=3
Camear Calibration 과정에서 위에서 언급한 Camera model의 parameters를 구해진다.
위에서 살펴봤듯이 DoF=11 이므로 Camera Calibration을 하기 위해선, 6개의 correspondances 가 최소로 필요함.
- 1개의 correspondance마다 2개의 식이 정의됨.
4. 같이 읽어보면 좋은 자료
[CV] Camera Model Parameter Estimation: $\underset{\textbf{x}}{\text{argmin }} \mathbf{x}^T A^T A \mathbf{x}$
Camera Model Parameter Estimation 문제를$$\underset{\mathbf{x}}{\text{argmin }} \mathbf{x}^T A^T A \mathbf{x} \\ \text{subject to } \ ||\mathbf{x}||=1$$조건부 최적화 문제로 유도하는 과정을 설명함.Camera Model Parameter Estimation의
dsaint31.tistory.com
https://jordicenzano.name/front-test/2d-3d-paradigm-overview-2011/camera-model/
Camera model
Introduction In this section I explain all necessary steps to obtain a mathematical camera model. Pinhole Camera – Image formation If we put a film directly in front of the object that we wan…
jordicenzano.name
https://kr.mathworks.com/help/vision/ug/camera-calibration.html
카메라 보정이란? - MATLAB & Simulink - MathWorks 한국
다음 MATLAB 명령에 해당하는 링크를 클릭했습니다. 명령을 실행하려면 MATLAB 명령 창에 입력하십시오. 웹 브라우저는 MATLAB 명령을 지원하지 않습니다.
kr.mathworks.com
'Programming > DIP' 카테고리의 다른 글
| [CV] Two View Geometry (0) | 2024.06.28 |
|---|---|
| [CV] Camera Model Parameter Estimation: $\underset{\textbf{x}}{\text{argmin }} \mathbf{x}^\top A^\top A \mathbf{x}$ (0) | 2024.06.23 |
| [Fitting] Hough Transform (0) | 2024.06.13 |
| [CV] Fitting (0) | 2024.06.13 |
| [DIP] Image Stitching (0) | 2024.06.13 |