Differentiable and Continuous
Function $f(x)$가 $x=a$에서 미분 가능하면: $p$
$f(x)$는 연속이다. : $q$
(▲ implication, 조건명제)
- $p \implies q$ 는 참(True)이나
- 이의 역(inverse)인 $q \implies p$는 거짓(False)임.
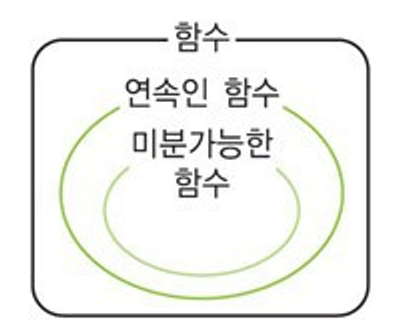
Example
- $f(x)=|x|$ : $x=0$에서 continuous하지만 미분가능하지 않음.
2023.06.22 - [.../Math] - [Math] Continuity (of Multivariate Function) and Contiguity
[Math] Continuity (of Multivariate Function) and Contiguity
Continuity (연속) 이란 If $S\subseteq \mathbb{R}^n$, then a function $f:S\to \mathbb{R}$ is continuous at $\textbf{a} \in S$ if $$\begin{equation}\label{cont.def} \forall \varepsilon >0, \ \ \exists \delta>0 \mbox{ such that if } \mathbf x \in S \mbox{
dsaint31.tistory.com
Differentiable의 조건 (Scalar Function)
- Function(함수)가 Continuous(연속)이어야 함.
- 만약 continuous(연속)이라면 좌미분계수 와 우미분계수가 같아야 함.
$$
\lim_{x \to a^-}\dfrac{f(x)-f(a)}{x-a}=\lim_{x \to a^+}\dfrac{f(x)-f(a)}{x-a}\\ \lim_{\Delta x \to 0^-}\dfrac{f(a+\Delta x)}{\Delta x}=\lim_{\Delta x \to 0^+}\dfrac{f(a+\Delta x)}{\Delta x}\\ \text{left derivative}=\text{right derivative}
$$
더 읽어보면 좋은 자료
2023.06.23 - [.../Math] - [Math] Differentiability of MultivariableFunctions
[Math] Differentiability of MultivariableFunctions
Differentiability $\textbf{f}:\mathbb{R}^n \to \mathbb{R}^m$ 이고, $\textbf{a} \in \mathbb{R}^n$이면서 $\textbf{f}$의 domain에 속한다고 하자. 이 때 $$ \underset{\textbf{x}\to\textbf{a}}{\lim} \frac{\textbf{f}(\textbf{x})-\textbf{f}(\textbf{a
dsaint31.tistory.com
https://dsaint31.github.io/math/math-week03/
[Math] Week 03
Limit and Continuity
dsaint31.github.io
'... > Math' 카테고리의 다른 글
| [Math] Probability 란: Probability, Odds, Likelihood (0) | 2024.04.17 |
|---|---|
| [Math] Variance and Standard Deviation: Measure of Dispersion (0) | 2024.04.17 |
| [Math] Derivatives of Exponential and Logarithmic Functions (0) | 2024.02.28 |
| [Math] Limits of Log and Exponential Functions (0) | 2024.02.28 |
| [Math] The Key Rules of Differentiation (0) | 2024.02.28 |