[LA] Existence and Uniqueness Theorem

Consistent Linear System

만약 Linear system이 consistent하다는 애기는 다음과 equivalent임.

• 해당 system의 augmented matrix에서 pivot column vector가 맨 오른쪽의 column이 되는 경우가 없음.
  • $\text{rank}(A) = \text{rank}(A|\textbf{b})$ 이 성립
• 이는 REF (row echelon form)로 augmented matrix를 표시할 때, $\textbf{0}^\top | b$ 와 같은 row가 없다는 애기임. (여기서 $\textbf{0}^\top$은 zero row vector로 bold체로 표시됨.)

---

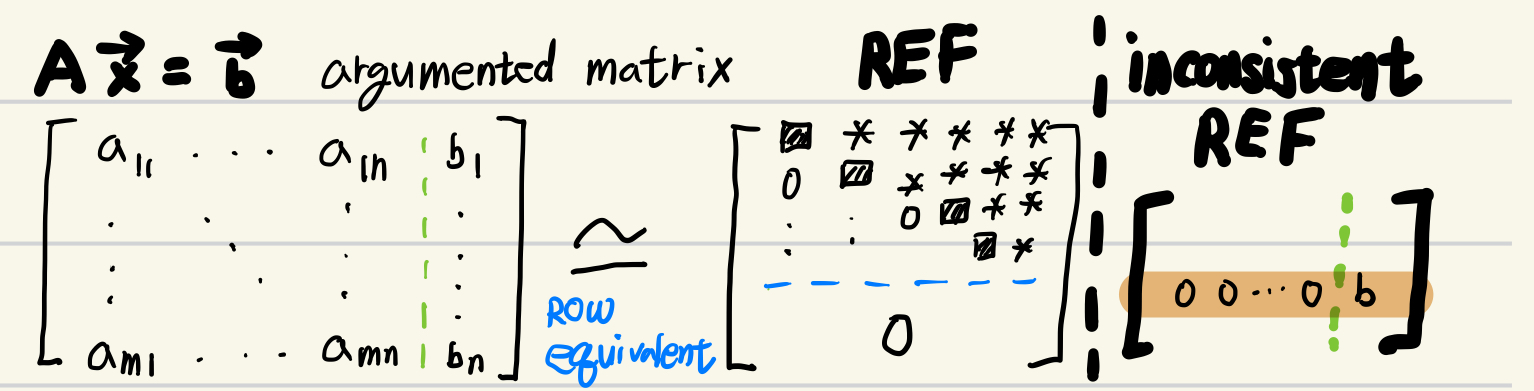
Linear system이 consistent 할 경우 다음 두가지 경우 중 하나임.

• unique solution을 가짐 : 이 경우 free variable이 없음. (즉, REF에서 all zero row가 없음.)
  • $\text{rank}(A) = \text{rank}(A|\textbf{b}) = n$
  • $n$ is the number of unknowns.
• infinitely many solution을 가짐 : 이 경우 free variable이 존재. (즉, REF에서 all zero row가 존재.)
  • $\text{rank}(A)=\text{rank}(A|\textbf{b}) < n$

참고로 pivot의 숫자가 바로 basic variable의 숫자임.

• basic variable: leading entry에 해당하는 variable로서
  • completely determined (특정 값으로 결정됨) 이거나
  • free varaiable이 존재하는 경우엔, 다른 variable들에 의해 값이 결정됨. leading variable이라고도 불림.
• free variable: REF에서 all zero row에 해당하는 variable임.

만약 $\text{rank}(A) < \text{rank}(A|\textbf{b})$ 인 경우는 inconsistent로 해가 존재하지 않음.

---

2024.02.17 - [.../Linear Algebra] - [LA] Pivot and Pivot Column

[LA] Pivot and Pivot Column

---

Example

다음과 같은 RREF (reduced echelon form)를 가지는 linear 시스템의 경우를 살펴보자.
$$\begin{bmatrix}
1 & 0 & -5 & 1 \\
0 & 1 & 1 & 4 \\
0 &0 &0 &0
\end{bmatrix}$$

위와 equivalent인 연립방정식 표현은 다음과 같음.
$$\begin{aligned}
x_1 - 5x_3 & = 1 \\
x_2 +x_3 &= 4 \\
0&=0
\end{aligned} $$

이 경우, pivot에 해당하는 $x_1$과 $x_2$는 basic variable이라고 불리며, 아닌 $x_3$는 free variable이 됨.

solution은 다음과 같이 표현된다.

$$\begin{aligned}
x_1 &= 1 + 5x_3 \\
x_2 &= 4-x_3 \\
x_3 & \text{ is free}
\end{aligned}$$

• 위와 같은 형태를 parametric description of solution sets이라고 함. 여기서 parameter는 $x_3$임.
• 주의할 것은 대수적 변환을 통해 $x_2$를 parameter로 한 parameter description도 가능하다는 점임.
• basic variable의 수가 2개이고 free variable이 1개라는 사실은 변하지 않으나 free variable은 $x_3$, $x_2$ 중 하나가 될 수 있음.

Solution에 대한 General form의 경우,
REF에서 leading entry(Pivot)를 통해 basic variable로 삼고,
all zero row로 free variable을 결정.

 

이 예제는 위에서 보였듯이 infinitely many solution을 가진 경우임.

---

같이 읽어보면 좋은 URLs

2024.02.17 - [.../Linear Algebra] - [LA] Pivot and Pivot Column

[LA] Pivot and Pivot Column

2024.02.16 - [.../Linear Algebra] - [LA] Linear Equation (선형 방정식) and Linear System 정리.

[LA] Linear Equation (선형 방정식) and Linear System 정리.

---