728x90
입력이 $x(t)$이고 최종출력이 $y(t)$인 parallel connection의 diagram은 다음과 같음.
- $h_1(t)$과 $h_2(t)$는 subsystem1과 subsystem2의 impulse response임.
- $y_1(t)$과 $y_2(t)$는 subsystem1과 subsystem2의 output임.
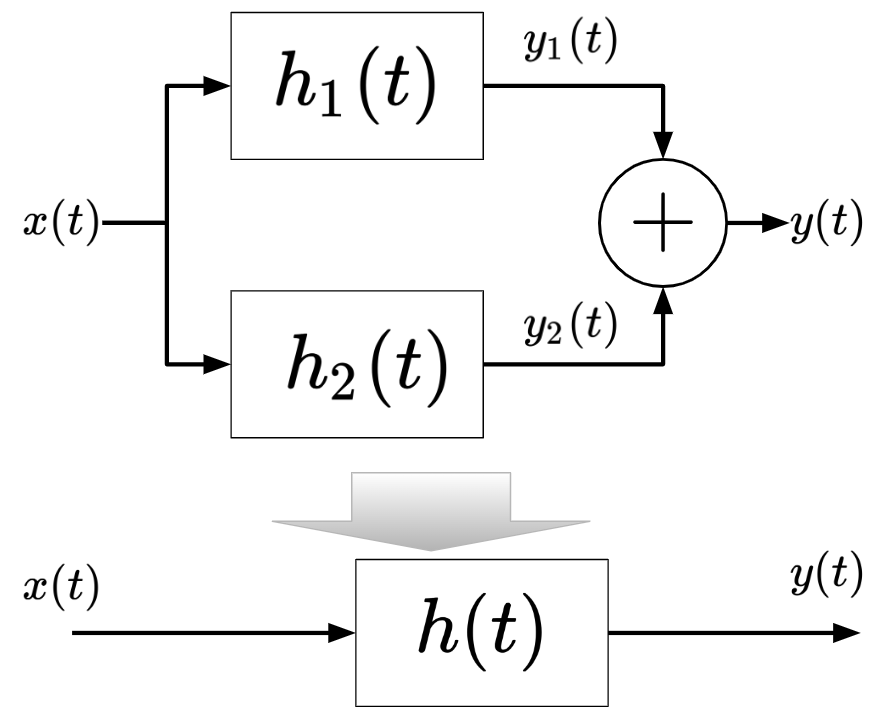
위와 같은 parallel connection system에서 impulse function이 입력되면 다음을 만족함.
- $x(t)=\delta(t)$
- $y_1(t)=h_1(t)$
- $y_2(t)=h_2(t)$
- $y(t)=h(t)=h_1(t)+h_2(t)$
즉, parallel connection의 경우 각 subsystem의 impulse response를 더함으로서 최종 impulse response가 구해짐.
위의 Parallel connection도 feed forward connection의 한 종류임.
여기서 다룬 모든 system들은 linear time invariant system임.
convolution과 impulse repsonse를 가지고 output을 구하는 것
자체가 이를 기반으로 이루어짐을 기억할 것.
반응형
'... > Signals and Systems' 카테고리의 다른 글
| [SS] BIBO Stable System (0) | 2023.08.22 |
|---|---|
| [SS] Feedback Connection (0) | 2023.08.22 |
| [SS] Cascade Connection (0) | 2023.08.22 |
| [SS] Differential Equation and Responses (zero-input, zero-state, natural, forced) w/o Laplace Transform (0) | 2023.08.22 |
| [SS] Differential Equation and Response (zero-input, zero-state, natural, forced) w/ Laplace Transform (0) | 2023.08.22 |