Tangent Vector
Tangent Vector 는 주어진 점에서 곡선 또는 곡면에 접하는 벡터로,
그 점에서의 곡선이나 곡면의 접선 방향을 나타냄.
이는 주로 매개변수화된 곡선 또는 곡면에서 정의됨.
물리 등에서 많이 사용되는 경우는
- Vector function $\textbf{r}(x)$, $\bf{r}: \mathbb{R}\to \mathbb{R}^n$, 의 Derivative(도함수)로써,
- 해당 derivative를 tangent vector라고 부름.
$$
\textbf{t}(x) = \textbf{r}^\prime(x)
$$
- Scalar function의 derivative는 tangent (접선, 기울기)가 됨.
- Multi-variate function (=multi-variable single-valued function, scalar field)의 derivative는 tangent plane이 됨.
vector function은 single-variable multi-valued function으로,
시간($x$, 독립변수)에 따른 관심대상의 위치($\textbf{r}(x)$)를 나타내는 함수 등을 예로 들 수 있다.
( 이 경우, 위치에 대한 derivative이므로 velocity vector가 바로 tangent vector임)
Tangent vector의 방향에 집중해야하는 경우엔 unit tangent vector를 사용함.
$$
\textbf{t}(x) = \frac{\textbf{r}^\prime(t)}{||\textbf{r}^\prime (t) ||_2}
$$
참고 : Tangent plane
tangent vector를 확장한 것이 tangnet plane임.
vector function 대신에, scalar field 에 대한 derivative.
scalar field $f(\textbf{x})$의 특정 point $\bf{x}_0$에서의 tangent plane $L_f(\textbf{x}_0)$은 다음으로 정의됨.
$$L_f(\textbf{x})=f(\textbf{x}_0)+\nabla f(\textbf{x}_0) (\textbf{x}-\textbf{x}_0)$$
Tangent Plane $L_f(\textbf{x})$는
$\bf{x}_0$ 근처에서의 $f(\textbf{x})$에 대한 local linear approximation임.
derivative를 통한 linear approximation에 대한 참고자료.
2023.06.23 - [.../Math] - [Math] Differentiation (or Differential, 미분)
[Math] Differentiation (or Differential, 미분)
"Differential을 구한다(Differentiation, 미분하다)" 는 derivative(도함수)를 구하는 것을 가르킴. Derivative(도함수)는 average rate of change의 limit인 instantaneous rate of change (←linear approximation의 slope)임. $$ \displays
dsaint31.tistory.com
tangent plane에 대해선 다음 동영상을 참고.
응용
$\textbf{r}(x)$가 $x$sec(초)에서의 관심대상의 position vector를 구하는 function 경우,
- $\bf{r}^\prime(x)$는 $x$ 시점에서의 tangent vector는
- velocity vector 가 된다. (시간당 위치의 변화율의 크기와 방향 = velocity vector)
예: 아래 그림에서 $T(t)$가 tangent vector로 velocity를 나타내며, $N(t)$는 velocity와 orthogonal인 acceration vector임.
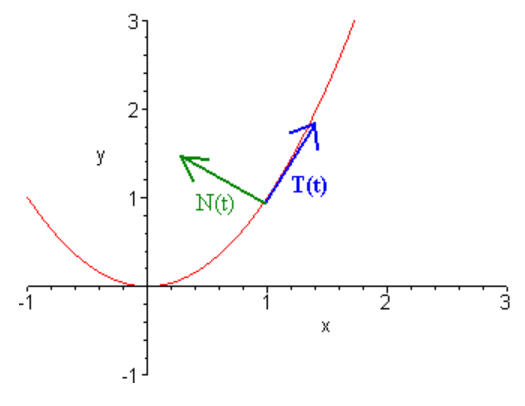
참고로, Scalar field에 대한 tangent plane를 구하는 방법은
여러 조건 하에서의 최대 최소를 찾는 optimizaion에서 많이 사용됨 (이 경우, scalar field가 loss function임).
- Lagrange method의 기본 원리이기도 함.
- 두 개의 level curvature가 접하는 접점에서는 각각의 gradient가 정확히 방향이 일치 (아니면 정반대방향)으로 평행하게 되며 이를 이용한 것이 Lagrange method임.
tangent plane은 복잡한 scalar field에 대한 일종의 local linearization을 수행하여 linear approximation을 제공하게 됨.
local에서 의미하듯이 특정 input vector에서의 해당 function의 값(e.g. loss function 값, 전하값 등)을 알면,
각 component의 partial derivative들을 통해 주변의 값들을 linear관계로 쉽게 구할 수 있음.
(엄밀한 의미에서 선형은 아니나 각 component에서 1차항만으로 처리가 가능함)
Normal Vector와의 관계
tangent vector에 대한 derivative가 바로 normal vector임.
normal vector와 tangent vector는 항상 orthogonal임.
$$
\textbf{n}(x) = \textbf{t}^\prime(x)
$$
읽어보면 좋은 자료.
https://dsaint31.tistory.com/entry/Math-Vector-2-Vector-Function#Derivative%--of%--Vector%--Function
'... > Math' 카테고리의 다른 글
| [Math] Upper Bound (상계) and Lower Bound (하계) (0) | 2023.07.05 |
|---|---|
| [Math] Lagrange Method or Lagrange Multiplier Method (0) | 2023.06.26 |
| [Math] Chain Rule (연쇄법칙) (0) | 2023.06.24 |
| [Math] Gradient (구배, 기울기, 경사, 경도) Vector (0) | 2023.06.24 |
| [Math] Directional Derivative (방향도함수) (0) | 2023.06.23 |