impulse

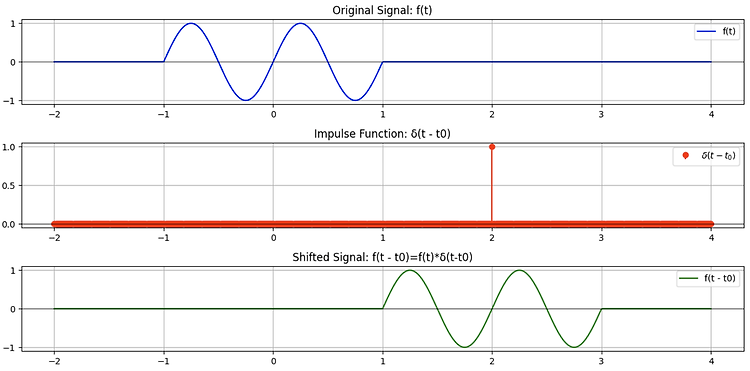
0. shifted impulse와 convolution은 결국 shifting 연산임$t_0$로 shifting을 시킨 impulse function $\delta(t-t_0)$과의 convolution은결국 같은 $t_0$만큼 signal을 shifting하는 것으로 볼 수 있음. $$f(t)*\delta(t-t_0) = f(t-t_0)$$ $*$ : convolution1. 증명$$ \begin{aligned} f(t)* g(t) &= \int^\infty_{-\infty} f(t-\color{red}{\tau}) g(\tau) d \tau \\ f(t)* \delta(t-t_0) &= \int^\infty_{-\infty} f(t-\tau) \delta(\tau - t_0) d \tau \\ &= \..

System의 impulse response는 다음을 의미한다.System의 input이 impluse signal인 경우의output을 해당 system의 impulse response라고 한다.LTI system에서 출력(정확히는 zero-state response)은 impulse response와 input signal의 convolution으로 표현 가능.LTI System에서의 Impulse response의 의미.LTI System의 impulse response를 파악했다면 다음이 성립함.System의 동작을 이해함. (정확히는 initial condition이 0인 경우의)System의 output 생성 메카니즘을 알 수 있음.임의의 input signal과 impulse response..

Impulse function (or Dirac delta function)은 이상적으로, 오직 한 점에서만 무한대의 값을 가지고,나머지에서는 0의 값을 가지며,적분시 면적인 1이 되는 함수다른 function을 분석하거나, system의 response를 측정할 때, Basis Function으로 사용됨.어떤 continuous function도 impulse function의 weighted sum으로 표현됨.Derivative (도함수) of Unit Step FunctionImpulse function은 unit step function의 derivative(도함수)라고 볼 수 있음. 유도.impulse function을 적분하면 다음이 성립함.$$\int^t_{\tau=-\infty} \delt..

다음과 같이 정의 되는 함수를 $\delta_\epsilon(t)$라고 하자. $$\delta_\epsilon( t ) =\left\{ \begin{matrix} 0 & ,t < -\frac { \varepsilon }{ 2 } \\ \frac { 1 }{ \varepsilon } & ,-\frac { \varepsilon }{ 2 } \le t