Rank

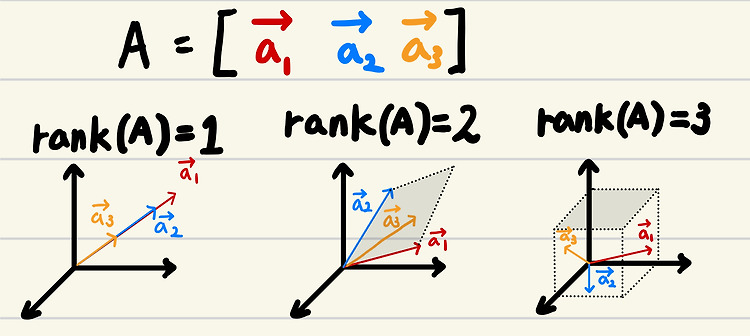
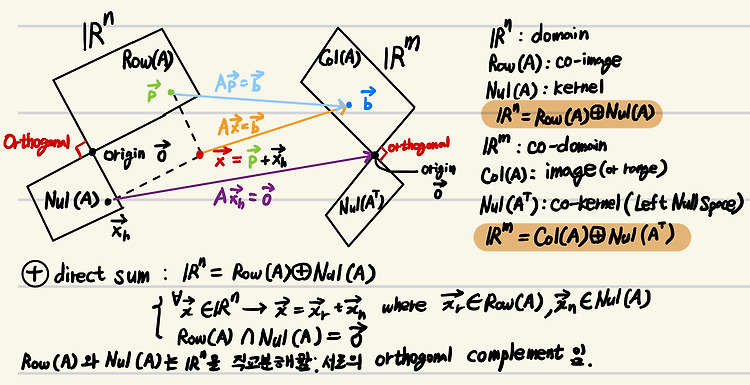
$A\mathbf{x} = \mathbf{b}$와 4개의 Fundamental Subspaces$A\mathbf{x} = \mathbf{b}$ 는 Linear System을 나타내는 Matrix Equation이면서, $\mathbb{R}^n$에서 $\mathbb{R}^m$으로의 Linear Transform을 나타냄.여기에서 4개의 중요한 Subspace 가 존재하며, 이를 이해하면 consistent에 대한 보다 깊은 이해 및 선형변환에서의 domain과 image등을 vector space로 파악할 수 있게 됨.0. Prerequisites더보기2024.10.28 - [.../Math] - [Math] Basis [Math] Basis기저(Basis)는 vector space (또는 function..

Null Space는 주로 matrix 에 관련된 맥락에서 사용되며,Linear Transform 의 맥락에서는 Kernel 이라고 불림.Definition : Null SpaceThe null space of an $m \times n$ matrix $A$, written as Nul$(A)$, is the set of all solutions of the homogeneous equation $A\textbf{x}=\textbf{0}$.In set notation, $$\text{Nul }(A) = \left\{ \textbf{x}:\textbf{x} \text{ is in }\mathbb{R}^n \text{ and }A\textbf{x}=\textbf{0} \right\}$$ Null Space 의 ..

Definition: Rank ◁ matrix 속성The rank of a matrix $A$, denoted by rank $A$,is the dimension of the column space of $A$.Matrix를 이루는 Column Vectors에서 Linearly Independent 인 것들의 수를 의미Row Space의 Dimension 의 경우를 강조하여 Row Rank라고 부르고,Column Space의 경우를 강조하여 Column Rank라고도 부르는 경우가 있으나,동일한 Matrix에 대해 이 둘은 같기 때문에 그냥 Rank라고 지칭하는게 일반적임. $m \times n$ Matrix $A$에서 다음이 성립. $$ \text{Column Rank}(A) \le n \\ \text..