728x90
Closed-form solution
💡 Solution(해)이 closed-form expression으로 주어진 것을 가르킴.
다음의 문장들 은 위와 같은 뜻.
- 방정식(equation)을 analytic method로 solution을 구할 수 있다.
- equation의 solution이 closed-form solution으로 표현된다
- equation의 solution을 analytic하게 표현할 수 있다.
- equation에 analytic solution이 존재.
일반적으로 어떤 방정식(equation)에 대한 solution을 구할 때, 해당 solution이 closed-form expression으로 구해질 때, close-form solution을 가진다고 말한다.
대표적인 예가 quadratic equation $ax^2+bx+c=0$의 solution임.
$$ x = \dfrac{-b\pm \sqrt{b^2-4ac}}{2a} $$
iterative method 및 수치해석적 기법의 중요성
- $f^\prime(x)$를 알 때 $f$ 를 찾는 미분방정식 (편미분방정식 포함)과 같은 경우 극히 일부만이 analytic solution(=close-form solution)을 가지고 있음. (← 공학수학에서 그 고생을 한 것들이 결국 일부라는 애기. 실제 비선형 미분방정식이나 coefficient가 변수인 경우 close-form solution은 없다고 봐도 됨)
- 때문에 현실적인 공학 문제에서 미분방정식 (편미분방정식 포함)을 풀기 위해선 수치해석적 기법이 절대적으로 필요함.
- 더불어, 통계의 cdf들의 경우도 대부분 테이블로 제공이 되는 이유도 close-form solution이 없기 때문이다.
수치해석이란
컴퓨터를 이용하여 수학적인 문제를 해결(equation의 solution, 정확히는 approximation of solution을 구하기)하기 위한 방법에 관한 학문.
Closed-form expression
In mathematics,
- a closed-form expression is a mathematical expression (표현식) that
- uses a finite number of standard operations. (←유한한 갯수의 연산 으로 표현됨)
It may contain
- constants,
- variables,
- certain well-known operations (e.g., + − × ÷), and
- functions (e.g.,
- n-th root,
- exponent,
- logarithm,
- trigonometric functions, and
- inverse hyperbolic functions),
but usually no (← 일반적으로 다음을 포함하지 않음.)
- limit, (극한)
- differentiation (미분), or
- integration (적분).
The set of operations and functions may vary with author and context. ← 이게 머리아픈 점.
Closed-form과 수학적으로 대비되는 개념은 바로
Open-form으로
- 쉽게 생각해서 유한한(finite)한 수학적 experssion이 불가 한 경우를 가르킴.
- 이 경우 대부분 iterative optimzation과 같은 수치해석적 기법을 이용한 approximation을 구하게 됨.
때문에 equation의 solution을 구하는 방법을 크게 analytic method와 iteratvie method로 구분하기도 함.
References
- (https://en.wikipedia.org/wiki/Closed-form_expression)
- (https://adnoctum.tistory.com/294)
- (http://wanochoi.com/?p=5061)
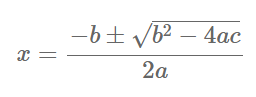
반응형
'... > Math' 카테고리의 다른 글
| [Statistics] Covariance vs. Correlation (0) | 2022.05.01 |
|---|---|
| [Statistics] Sample Point Method (0) | 2022.05.01 |
| Normal Equation : Vector derivative 를 이용한 유도 (0) | 2022.04.28 |
| One sample t-test : The Moon Illusion (0) | 2022.04.27 |
| Chi Square : Independence Test (Analysis of Contingency Table) (0) | 2022.04.25 |