[SS] Fourier Series (푸리에 급수)
Fourier Series
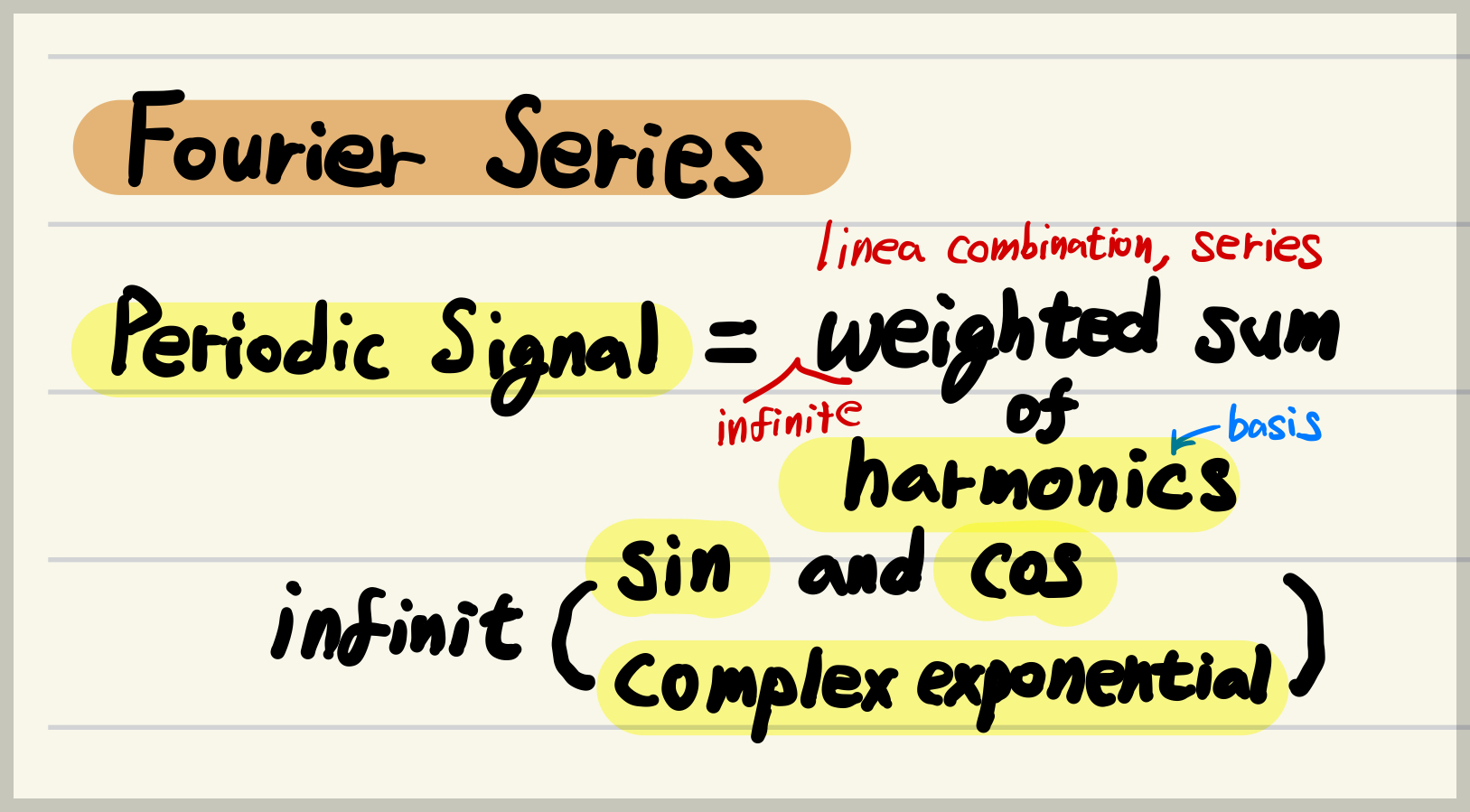
Fourier Series는
- periodic signal (주기함수로 표시됨)을
- 해당 signal의 fundamental frequency(기본주파수, $\Omega_0=\frac{2\pi}{T}$)의 정수배 frequency를 가진 harmonics를 basis로 삼아
- 이들을 각각의 amplitude와 phase를 적절히 조절(=Fourier Series Coefficient를 조절)하고,
- 이들을 더함(series)으로서 표현하는 방법임.
2024.10.28 - [.../Math] - [Math] Basis
[Math] Basis
기저(Basis)는 vector space (또는 function space) 에서 모든 요소(Element)를 나타내기 위해 필요한 최소한의 (선형)독립적인 요소 집합(vector set or function set).Basis (기저)는 벡터 공간 (또는 함수 공간)의 구
dsaint31.tistory.com
2024.02.16 - [.../Linear Algebra] - [LA] linear combination
[LA] linear combination
Linear Combination이란linear equation 에서 variables이 scalars $x_n$가 아닌 vectors $\mathbf{x}$로 대체한 형태 .scalar를 component가 1개인 vector라고 생각할 수 있으므로, linear equaiton의 (right side의) 일반화 라고 봐도
dsaint31.tistory.com
2023.06.16 - [.../Signals and Systems] - [SS] Periodic Signal (주기신호): Fundamenta Frequency 등에 대해 확인.
[SS] Periodic Signal (주기신호)
정의 Signal이 일정한 간격($T$, period, 주기)을 가지고 값과 형태가 동일하게 반복되는 경우, 해당 signal을 주기성(periodicity)을 가진다고 하고 periodic signal이라고 칭함. aperiodic signal은 주기성을 가지
dsaint31.tistory.com
2025.04.27 - [.../Signals and Systems] - [SS] Harmonic (조화파) 이란?
[SS] Harmonic (조화파) 이란?
Harmonic 이란?harmonic 은 periodic signal 을 구성하는 기본 요소로서,Fundamental Frequency (기본 주파수, $\Omega_0$) 의 정수배에 해당하는 주파수 성분, $k\Omega_o (k \text{ is int})$을 의미. 2023.06.16 - [.../Signals and S
dsaint31.tistory.com
periodic signal $\tilde{x}(t)$를 Exponential Fourier Series (첫째 식)로 나타낸 것과, 이 식에서 $k$번째 harmonic에 대한 Exponential Fourier Series Coefficient $X_k$ (두번째 식)를 나타낸 것은 다음과 같음:
$$\tilde{x}(t)=\sum^\infty_{k=-\infty}X_k e^{jk\Omega_0 t} \\ X_k=\frac{1}{T}\int^{\frac{T}{2}}_{-\frac{T}{2}}\tilde{x}(t)e^{-jk\Omega_0 t }dt$$
- Fourier coefficient $X_k$는 complex number로 k-th harmonic의 amplitude와 phase를 결정.
- $X_k = |X_k|e^{j\angle X_k}$로 polar coordinate form으로 표현되며,
- $|X_k|$가 k-th harmonic의 amplitude이며
- $\angle X_k$가 k-th harmonic의 phase임.
- 또한 $X_k = X_{-k}^*$로 complex conjugate가 성립: Hermitian Symmetry
Fourier Series는 periodic signal을 $X_k$ 로 나타내는데,
이는 complex number이므로 amplitude spectrum과 phase spectrum으로 표현됨.
간단히 말하면,
Fourier Series는
periodic signal 을
해당 신호의 무한 개의 harmonics에 대한 weighted sum
(=infinite series)으로 표현하는 방법임.
2023.07.21 - [.../Math] - [Math] Sequence (수열) and Series (급수)
[Math] Sequence (수열) and Series (급수)
Sequence수열, 열 이라고 불림.numbers나 objects 들이 순서를 가지고(ordered) 나열된 것을 가리킴.order(순서)가 의미를 가지며, (order가 다르기 때문에 )중복이 허용이 된다.대표적인 예로 arithmetic sequence(
dsaint31.tistory.com
2022.09.21 - [.../Signals and Systems] - [SS] Orthogonal Function: Complex Exponential Function
[SS] Orthogonal Function: Complex Exponential Function
0. Fourier Transform의 Basis Function지수 함수 (정확히는 복소지수함수)는 대표적인 orthogonal function으로 Fourier transform의 basis로 사용이 된다.구간 $T$에서 Orthogonal function인 경우, 해당 구간에서 inner product
dsaint31.tistory.com
Fourier Series의 특징.
이는 다음의 특징을 가짐:
- 특정 신호에서 특정 주파수 성분(=하나의 harmonic에 대응)이 얼마나 기여하는지를 분석할 수 있음.
- 복잡한 periodic signal (or periodic function)을 sine과 cosine의 weigthed sum으로 표현함.
- 즉, periodic signal의 또 다른 representation(표현법)이라고도 볼 수 있음.
2022.04.19 - [.../Physics] - [Physics] A periodic representation of wave : sin and cos
[Physics] A periodic representation of wave : sin and cos
$\sin$(정현), $\cos$(여현) : 주기신호나 wave등을 나타내는데 사용되는 기본적인 함수. $$ y(t)= A \sin(\omega t + \theta) \\ y(t) = A \cos (\omega t + \theta)$$ $T$ : period (주기), 단위 : sec. Time to complete one vibration $\om
dsaint31.tistory.com
기존의 impulse function을 basis로 한 linear combination 표현 대신에,
- fundamental frequency의 정수배에 해당하는 harmonics에 대한 cosine과 sine (또는 이를 조합한 complex exponential)을
- basis로 하는 linear combination 표현을 가능하게 해줌.
단, periodic function 에 대해서만 사용가능하다는 단점이 있어서
이를 개선한 Fourier Transform이 보다 많이 사용됨.
2023.12.07 - [.../Signals and Systems] - [SS] Fourier Analysis: 4가지 Fourier Transform 비교
[SS] Fourier Analysis: 4가지 Fourier Transform 비교
Fourier representation(표현)!!signal을 frequency와 대응관계인 (complex)sinusoidal signal(복소정현파)의 weighted sum 으로 표현달리 말하면(complex)sinusoidal signal을 basis function으로 삼아이들의 linear combination으로 표
dsaint31.tistory.com
Trigonometric Fourier Series and Complex Exponential Fourier Series
다음은 Trigonometric Fourier Series를 나타냄:
$$\begin{aligned} \tilde{x}(t) &= a_0 + \sum^\infty_{k=1} \left[ a_k \cos k\Omega_0 t + b_k \sin k\Omega_0 t \right], T=\frac{2\pi}{\Omega_0} \\ a_0 &= \frac{1}{T}\int^{t_0+T}_{t_0}\tilde{x}(t) dt \\ a_k &= \frac{2}{T}\int^{t_0+T}_{t_0}\tilde{x}(t) \cos k\Omega_0 t dt, k=1,2, \dots \\ b_k & = \frac{2}{T}\int^{t_0+T}_{t_0} \tilde{x}(t) \sin k\Omega_0 t dt, k = 1,2, \dots \end{aligned}$$
- Trigonometric Functions를 basis로 사용하여 표현할 경우,
- Fourier Series Coefficients $a_k, b_k$이 real number 가 된다는 장점을 가짐.
- k-th Hormonic의 실제 amplitude $A_k$ 은 다음이 성립: $A_k = \sqrt{a_k^2 + b_k^2}$
- k-th Harmonic의 phase $\phi_k$은 다음이 성립: $\phi_k = \tan ^{-1} \left( \frac{-b_k}{a_k} \right)$
앞서의 basis인 complex exponential $e^{jk\Omega_0 t}$와 cosine과 sine의 관계는 다음과 같음.
$$\begin{aligned}a_k \cos k\Omega_0 t + b_k \sin k \Omega_0 t &=a_k \frac{e^{jk\Omega_0 t}+d^{-jk\Omega_0 t}}{2} + b_k \frac{e^{jk\Omega_0 t}-e^{-jk\Omega_0 t}}{2j} \\ &= \frac{a_k -j b_k}{2}e^{jk\Omega_0 t} +\frac{a_k +j b_k}{2}{e^{-jk\Omega_0 t}} \\&= X_k e^{j k \Omega_0 t} +X_{-k} e^{-j k \Omega_0 t} \end{aligned}$$
- trigonometric bais에서는 양수의 2개 항들 (left-hand side)이
- compelx exponential 에서는 1개의 음수항과 1개의 양수항 (right-hand side)으로 변함.
- trigonometric basis와 complex exponential bais의 관계는 Euler identity임.
trigonometric functions 와 complex exponeital functions 모두 orthognal function 이며,
특정 주파수를 대표하기 때문에 Frequency Representation의 basis function으로 자주 사용됨.
Euler Identity는 다음 url을 참고:
2023.10.25 - [.../Math] - [Math] Euler’s Constant (자연상수, 오일러 상수) / Euler's Identity
[Math] Euler’s Constant (자연상수, 오일러 상수) / Euler's Identity
가장 유명한 irrational number(무리수)로 대략 2.718281... 정도의 크기를 가짐.가장 유명한 극한값이기도 함: $0,1,\pi,e$ 는 가장 중요한 4가지 수라고도 불릴 정도이며 공학자에게 $e$는 정말 정말 중요함
dsaint31.tistory.com
주기신호의 Frequency Representation: Fourier Series
Fourier Series는 결국 주기신호의 주파수 성분을 잘 나타내는 표현이다.
특정 주파수 성분은
- Orthoganl 성질을 가지면서 주기성(=나타내고자 하는 주파수)을 가진 함수(=Fourier Series에선 Harmonic)를
- Basis Function (기저함수)로 삼고, 이에 대한 complex coefficient로 표시함.
- 이 complex coefficient는 해당 주파수의 amplitude와 phase에 해당함.
이를 쉽게 구하기 위해 orthogonal periodic function을 사용(신호에 inner product를 하면 쉽게 complex coefficient를 구하므로)
- 일반적으로 Trigonometric function (sine and cosine) 또는 이들을 Euler identity를 통해 변환한 Complex Exponential Function이 사용됨.
2023.10.04 - [.../Signals and Systems] - [SS] Orthogonal function: inner product가 0
[SS] Orthogonal function: inner product가 0
1. Orthogonal이란어떤 두 개의 대상이 Othrogoanl (직교)하다는 의미는 (보통 대상은 function 또는 vector임)해당 두 대상의 inner product의 결과가 0이라는 의미이며, 두 대상이 각각에 대해 공유하는 component
dsaint31.tistory.com